Landlocked Pentominoes, Revisited
Just over twenty years ago I wrote an article on this website about 6x10 pentomino cofigurations with "landlocked" pieces. It is known, by exhaustive search through all 6x10 solutions, that you can have, at most, four pieces that are landlocked.Recently, however, I thought about that article and wondered what would happen if you removed the restriction of the final configuration being a 6x10 rectangle? What if you allowed the final arrangement of pieces to be any, possibly very irregular, shape? How many pieces could you landlock within that arrangement?
After about a half hour of experimentation, I have discovered this configuration that has six of the pentominoes landlocked:
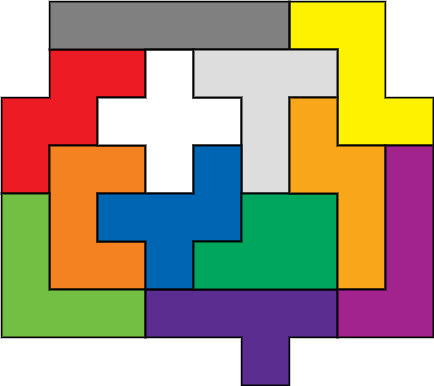
Note, that I am assuming a couple of things about the construction of my blobs:
- The shape, however irregular it may be, should have no interior holes. It should be solid.
- In order for a piece to be landlocked, it must be fully obstructed from the edge of the blob, even at its corners. In other words, if a piece is landlocked, by "stepping" in any direction off of it (including diagonally) you must visit another piece before reaching the edge of the whole blob of pieces.
This solution is not unique. For example, the F- and X-pentominoes could be easily swapped in position, and the Y-pentomino could be flipped.
However, I'd bet that it is not possible to improve to seven landlocked pieces. If anyone can find such an arrangement (or actually prove that six is the maximum), please do contact me.
Doubly Landlocked
And here's another variation. Is it possible to construct a configuration in which a piece is "doubly landlocked". Meaning, that if you start at that piece and step off of it (including diagonally), you must visit at least two other pentominoes before reaching the edge of the blob.Here is a configuration I have found in which the X-pentomino is doubly landloked:
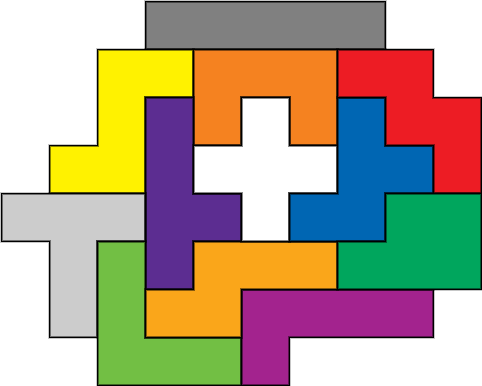
Is it possible to doubly landlock one of the other pentominoes?
Is it possible to doubly landlock two (or more) pieces at once?
I do not yet know...
- Eric Harshbarger, 2 April 2025